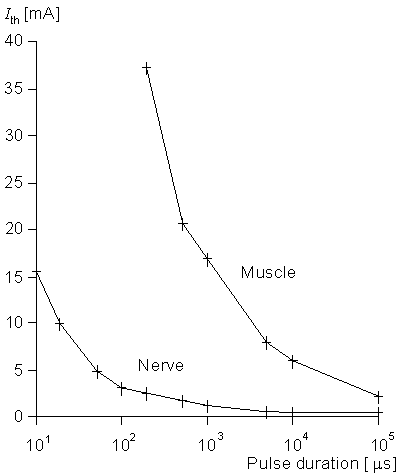
There are a growing number of documented scientific therapeutic uses of electricity (Seligman, 1982). In most cases, although the mechanism is not always clearly understood, it appears to arise as a consequence of the depolarization or hyperpolarization of excitable cell membranes resulting from the applied currents. Other mechanisms that appear to be sometimes involved include thermal (heating) and neurohumoral effects.
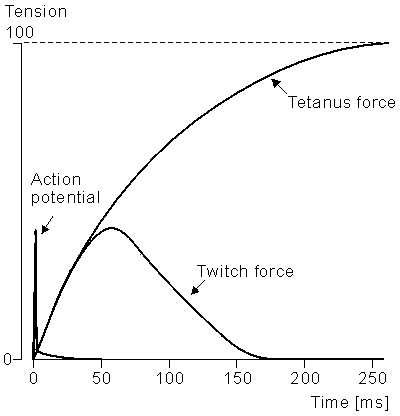
 Functional electric stimulation is a very straightforward application for the therapeutic use of electricity. Another area in which electrotherapy may be applied is in the electric stimulation of cardiac tissue, including cardiac pacemakers and cardiac defibrillation. These topics are discussed in the next two chapters.
Functional electric stimulation is a very straightforward application for the therapeutic use of electricity. Another area in which electrotherapy may be applied is in the electric stimulation of cardiac tissue, including cardiac pacemakers and cardiac defibrillation. These topics are discussed in the next two chapters.
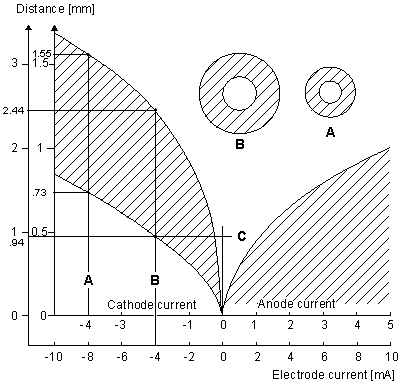
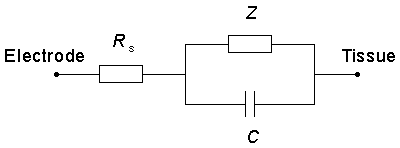
 Since the electric stimulation of biological tissues requires the use of electrodes, any practical study should include consideration of electrodes and electrode-tissue interaction. The mechanical properties of electrodes are important particularly with respect to implants whose lifetime is measured in years. Since the flow of electricity from the electrode (where electrons carry the charges) into the tissue (where ions carry the charges) may involve an electrochemical reaction, this area must be carefully studied as well. Consequently, several sections of this chapter are devoted to these electrode characteristics.
Since the electric stimulation of biological tissues requires the use of electrodes, any practical study should include consideration of electrodes and electrode-tissue interaction. The mechanical properties of electrodes are important particularly with respect to implants whose lifetime is measured in years. Since the flow of electricity from the electrode (where electrons carry the charges) into the tissue (where ions carry the charges) may involve an electrochemical reaction, this area must be carefully studied as well. Consequently, several sections of this chapter are devoted to these electrode characteristics.
  | (21.1) |
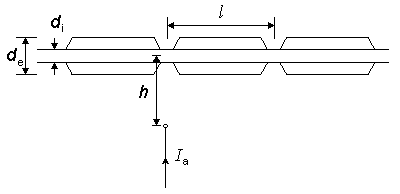
| where | ri | = axial intracellular resistance per internodal length [kW/l] |
| ri | = intracellular resistivity [kW·cm] (chosen as 0.1 kW·cm) | |
| l | = internodal length [cm] | |
| di | = axon diameter (internal myelin diameter) [cm] |



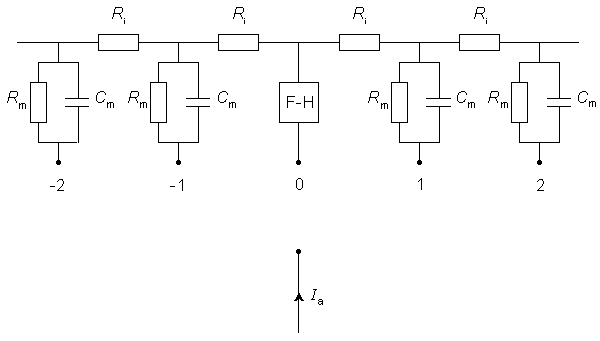





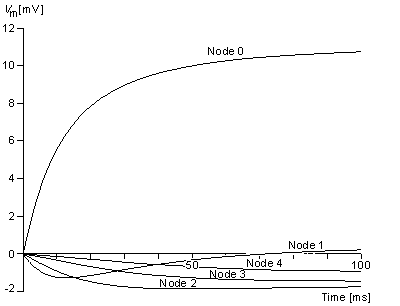
 15 ms and that for t >15 ms occur at different nodes!
15 ms and that for t >15 ms occur at different nodes!
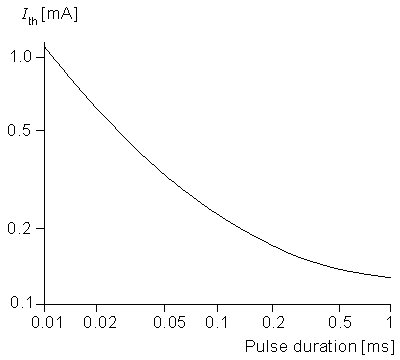
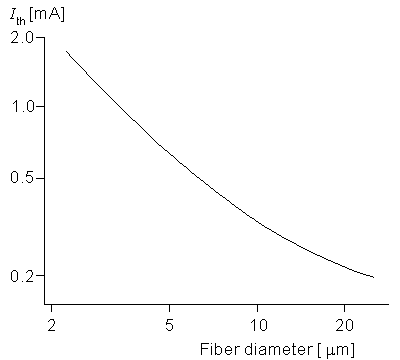




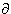 2Vo/
2Vo/ 0, hence less satisfactory for myelinated fibers where Dx = l )
0, hence less satisfactory for myelinated fibers where Dx = l )



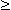 h /
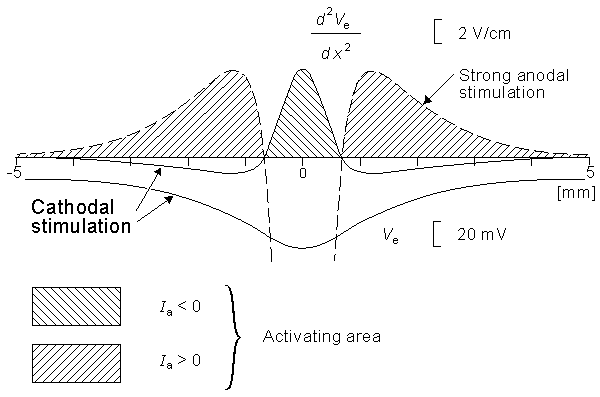
h /  2 (hyperpolarization is produced for | x |
2 (hyperpolarization is produced for | x |